Go Paradoxes
British Go Journal No. 63. November 1984. Page 20.
Andrew Grant
The Pitfalls of Endgame Counting.
Look at the moves in Dias 1 and 2. Which is bigger? Or are they equally big? Try counting the value of each.
Dia 1 Move A.                             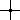                           
| |
Dia 2 Move B. |                                                     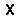   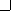
|
The normal methods of endgame counting can be used to show that move A is worth 10 points in gote, whereas B is worth 9½ points, also in gote. (This value is arrived at by averaging Black and White 'X'; it is assumed to be too small to be played immediately.
Dia 3 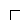                                                                                
| |
Dia 4 |                                                                                 
|
So A is the bigger move, isn't it? Well maybe not. Look at Dia 3. Should Black play A or B? Dia 4 shows what happens if he plays the 'larger' move first; he loses by one point. If, however, he starts with the 'smaller' move, he wins by one point, as in Dia 5.
Dia 5                                                                                 
| |
Dia 6 | 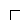   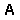                                                                     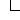        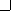
|
It seems, then, that the 'smaller' move, B, is actually two points better tan the 'larger' move, A. But let's just check our conclusion in another position (Dia 6) where there is another move added.
This time Black starts with B, but as Dia 7 shows, he loses by one point. If however he starts with A as in Dia 8, he wins by two points
Dia 7                                                                                 
| |
Dia 8 |                                                                                 
|
There is something else worth noticing in Dias 4, 5, 6 and 7, though; in each case the side which makes the final move wins the game. This is not just coincidence; it is a strategic principle of great importance in the late endgame, and it can, as here, be more useful than orthodox endgame counting.
Armed with this knowledge, try working ut the result if White has the first move in Dias 3 and 6 (give Black a 3 point komi). Should he play on the upper side, or on the lower side, or go for the last move? Is White's best starting point the same as Black's? These questions are left to the reader.
which is one of a series of back issues now available on the web.
If you have any comments, please email the webmaster on web-master AT britgo DOT org.
